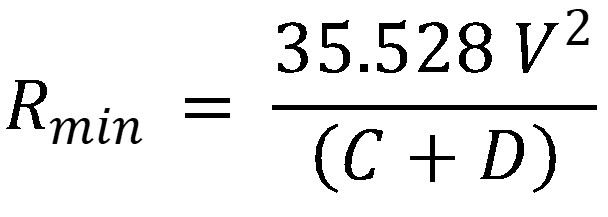
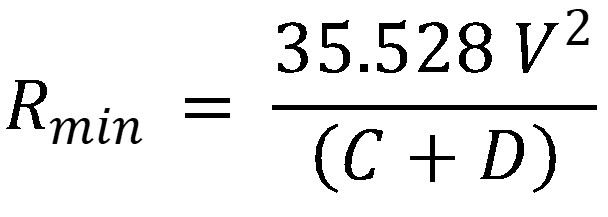
where:
Rmin = Minimum curve radius (m)
V = Line speed (mph)
C = Rail cant (mm)
D = Maximum cant deficiency (mm)
This is a commonly used formula, differing only in the units of measurement (mph or km/h). One such public source is here.
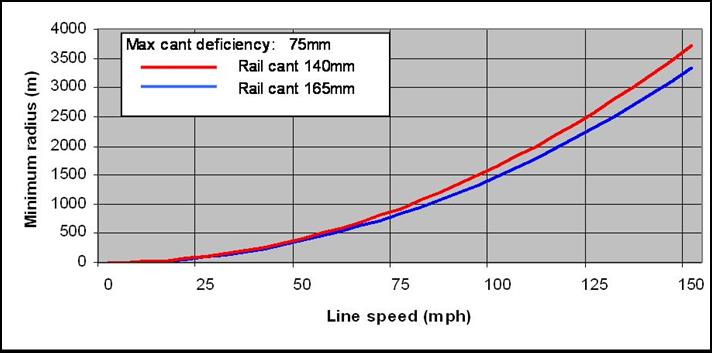
Rail cant is the amount of banking, or super-elevation of the outer rail above the inner rail. At equilibrium speed, the cant is such that the combined forces of the reaction to the train’s weight and its lateral acceleration are at right angles to the canted track (i.e. passengers’ soup will appear to stay level in the bowl). If a train travels faster than the equilibrium speed, passengers will feel a force towards the outside of the curve; if it travels more slowly, they will feel a force towards the inside of the curve. If the train stops on the curve, they will feel a sideways force caused by the tilted track. Cant deficiency is the amount by which the rail cant differs from that which would cause the train speed to be the equilibrium speed. Rail designers place limits on cant deficiency, both for passenger comfort and to limit rail wear. The typical maximum cant deficiency is 75mm.
Maximum rail cants differ with different line specifications and with different national design standards. However, typical ranges are 140mm – 165mm. The Airdrie – Bathgate technical feasibility report adopted a rail cant of 150mm.
The EISL proposals have used a rail cant of 165mm.
Figure 36 shows the minimum curve radius as a function of line speed for rail cants of 140mm and 165mm.